Consider a space station moving in a circular orbit around some massive body (a planet, a star, or perhaps a black hole), “tidally locked” so that it always keeps the same face towards whatever it’s orbiting. At the centre of the station objects will be weightless, but away from the centre how will they move? Or if they are kept from moving by appropriately oriented “floors”, what weight will they experience? These seem like very simple questions, but it turns out that they involve a lot of interesting physics, and can even reveal whether or not the station is in an orbit where relativistic effects come into play, without the need to make observations of anything outside the station itself.
An overview of the situation, with lots of illustrations, is given in this companion page. Here, we give a detailed mathematical treatment.
Since the station is much smaller than the size of its orbit, we can treat all forces as linear in the distances from the centre. This means it’s possible to completely describe the motion of all test particles in terms of the tidal stretching or squeezing in each of three orthogonal directions, along with the rate of spin experienced by the station. The tidal accelerations will grow linearly with distance from the centre of the station, so we will describe them as accelerations per unit distance. The centrifugal force due to spin also grows linearly with distance from the centre, within the plane orthogonal to the spin axis (which will be the same as the plane of the orbit). At first glance it might then seem that we can simply absorb the effects of spin into two of the tidal accelerations, but that would only account for centrifugal force; there is also the Coriolis force that is experienced by particles moving within the same plane, and which depends on their velocity in that plane rather than their position.
We will label the directions within the station as follows:
| Label for direction | Meaning | Name in Incandescence | Coordinate for measuring distance from centre |
|---|---|---|---|
| r | Outwards, away from central body | sard | x |
| –r | Inwards, towards central body | garm | –x |
| φ | Along direction of orbital motion | rarb | y |
| –φ | Opposite direction to orbital motion | sharq | –y |
| z | “Above” plane of orbit (orbit looks counter-clockwise from above) | shomal | z |
| –z | “Below” plane of orbit (orbit looks clockwise from below) | junub | –z |
We have used r and φ to label the radial and orbital directions, because these letters make the meaning of the directions in the context of the orbit very clear, but because r is used for distance from the central massive body and φ is an angular coordinate, we need to introduce coordinates x and y which measure distances in the r and φ directions from the centre of the station itself.
We will call the pure tidal accelerations ar, az and aφ, with the sign convention that tidal stretching is positive, and tidal squeezing is negative. In other words, given that moving away from the centre of the station in the positive r direction gives rise to an acceleration in the same direction, ar will be positive. In the absence of spin, this definition would mean d2x/dt2 = ar x, etc., but of course the equations for x and y will need to be modified to take account of spin.
We will call the angular velocity of the station’s spin (which is around the z-axis), ωs. In Newtonian mechanics this can really only have one meaning, but in general relativity we need to be clear and state that it is the rate (measured within the station) at which directions fixed with respect to the structures of the station rotate, compared to parallel transport. This can be discovered experimentally by looking at the rate at which a gyroscope precesses relative to the station walls: the gyroscope is taken as fixed, and the station is taken to be rotating with the same rate in the opposite direction.
We will call the net accelerations per unit distance, including the effects of centrifugal force, Ar, Az and Aφ, where:
Ar = ar + ωs2
Az = az
Aφ = aφ + ωs2
Now, it’s clear that particles that share exactly the same circular orbit as the station’s centre of mass, but are simply displaced a short distance along the orbit, will remain fixed relative to the station. So we will always have:
Aφ = aφ + ωs2 = 0
aφ = – ωs2
The remaining, non-zero net accelerations (Ar > 0, Az < 0), along with the Coriolis force, will determine the motion of anything free-falling within the station, and the weight felt by anything that is not in free fall. For a particle in free fall we have the differential equations:
d2x/dt2 = Ar x + 2 ωs dy/dt
d2y/dt2 = –2 ωs dx/dt
d2z/dt2 = Az z
In general, solutions to this set of equations will be unbounded, but there are two families of bounded, periodic solutions. One is fairly obvious:
x = 0
y = B
z = C cos(ωz t)
where B, C are any constants and ωz2 = –Az
This describes a particle oscillating back and forth across the plane of the orbit. We can make a particle execute this motion by placing it at rest at x=0, y=B, z=C, and releasing it. The other solution is a little less obvious:
x = D sin(ωr t)
y = 2 D (ωs / ωr) cos(ωr t) + B
z = 0
where B, D are any constants and ωr2 = 4 ωs2 – Ar
This describes a particle moving in an ellipse that is centred on the line x=0, the line of the station’s orbit (the “Null Line” in Incandescence). How do we get a particle to move like this? When t=0, we have x=0, dx/dt = D ωr, while y can be anything by choice of B, and dy/dt=0. So by firing a particle in the r-direction from the Null Line, we can guarantee that it executes this kind of cyclic motion.
From the perspective of orbital motion, the particle is travelling along a non-circular orbit which periodically returns it to the same relationship with the station; however, in general (in the relativistic cases) that period won’t be the same as the period of the station’s orbit. The particle’s orbit represents a slight perturbation to the station’s orbit that nonetheless remains close to it, an indication that the orbit is stable. But note that if Ar ≥ 4 ωs2, the motion ceases to be periodic, implying that the station’s orbit ceases to be stable!
The qualitative behaviour of various test particle trajectories and the relationships between their periods will depend on ratios between the three parameters, Ar, Az and ωs, so if we are concerned with such relationships rather than absolute time scales the number of parameters is reduced to two. Furthermore, there is an equation, ar + az + aφ = 0, that arises from the gravitational physics of tidal accelerations, and reduces the number of independent ratios to just one. So every dimensionless fact that these test particle motions can reveal by themselves (without reference to clocks or rulers calibrated by some non-gravitational process) can be encapsulated in a single number. For example, we have seen that if Ar / ωs2 ≥ 4, the station’s orbit is not stable. But we can answer the same question about the orbit’s stability by looking at another ratio, such as Ar / Az, the ratio between the net accelerations. It’s easy to show that:
Ar / ωs2 = 2 (Ar / Az) / [1 + Ar / Az]
Ar / Az = (Ar / ωs2) / [2 – Ar / ωs2]
and so the orbit will become unstable if Ar / Az ≥ –2.
We will now find explicit formulas for Ar, Az, and ωs in three cases: Newtonian gravity, the Schwarzschild geometry around a non-rotating black hole, and the Kerr geometry around a rotating black hole. In everything that follows, we will adopt units where the speed of light, c, and the gravitational constant, G, are equal to 1.
The station orbits the central mass M with an orbital angular velocity, ωorbit, such that orbital centrifugal acceleration balances gravitational acceleration:
ωorbit2 r = M / r2
ωorbit2 = M / r3
But in the Newtonian case, the fact that the rotation is tidally locked implies that the station’s rate of spin is exactly the same as its orbital angular velocity:
ωs = ωorbit = √(M / r3)
We can find Az = az easily by either of two approaches. The first is to note that by the spherical symmetry of the gravitational field, az = aφ, and since aφ = – ωs2 we have:
Az = az = aφ = – ωs2 = – M / r3
The second is to note that, again by spherical symmetry, a particle displaced in the z direction will move in an inclined orbit with the same period as that of the centre of the station, and for small displacements this will look just like simple harmonic motion with a “spring constant” equal to minus the square of the angular frequency of the motion. In other words, if:
z = C cos(ωorbit t)
then
d2z/dt2 = – ωorbit2 z
But by definition, d2z/dt2 = Az z, giving us the same result for Az.
We find ar by taking the derivative of the gravitational acceleration:
ar = d(–M/r2) / dr = 2 M / r3
Ar = ar + ωs2 = 3 M / r3
Note that ar + az + aφ = 0, a result that turns out to hold in general relativity as well as it does in Newtonian physics. In the novel Incandescence, this is known as “Zak’s principle”.
We have a ratio for the net accelerations of:
Ar / Az = –3
If we compute ωr, the angular frequency for the periodic motion we get if we fire a particle radially from the Null Line, we find:
ωr2 = 4 ωs2 – Ar = 4 M / r3 – 3 M / r3 = M / r3
This is the same as the other angular frequencies: that of the orbit, and the station’s spin. Another way we can think of ωr is in relation to the “effective potential” for a particle orbiting the central mass. A particle in a circular orbit of radius r0 has angular momentum per unit mass of:
J = ωorbit r02 = √(M r0)
The angular velocity of a particle with the same angular momentum, but not necessarily in a circular orbit, is:
ω(r) = (J / r2) = √(M r0) / r2
The energy per unit mass of this particle is:
E = – M / r + (1/2) (dr/dt)2 + (1/2) (r ω(r))2
= – M / r + (1/2) (dr/dt)2 + (1/2) (M r0) / r2
The second term is the kinetic energy (per unit mass) due to radial motion, Kr, so we have E = Kr + V(r), where:
V(r) = – M / r + (1/2) (M r0) / r2
So the radial motion of the particle will correspond to motion in this potential V(r). The first two derivatives of V(r), evaluated at r=r0, are:
dV(r)/dr|r=r0 = 0
d2V(r)/dr2|r=r0 = M / r03
So locally the potential well will look like a harmonic oscillator potential with a “spring constant” of M / r03, and a particle perturbed slightly from a circular orbit at r=r0 will undergo oscillations with a frequency whose square is equal to M / r03.
In the Schwarzschild geometry around a non-rotating black hole, the Schwarzschild r coordinate is defined so that the circumference of a circle of constant r, as measured by someone at rest with respect to the black hole, is 2πr. Far from the hole, changes in the r coordinate correspond to proper distances, but in the highly curved geometry close to the hole, the actual distance between, say, circular orbits with two different r values r1 and r2, will be greater than |r1 – r2|.
The hole’s event horizon is at r=2M. For objects with rest mass, circular orbits only exist for r > 3M (though photons can orbit at r = 3M), and those orbits are only stable for r > 6M.
The proper angular velocity of a circular orbit, as measured by a clock moving with the orbiting body, is given by:
ωorbit2 = M / [r2 (r – 3M)]
which is faster than the Newtonian angular velocity for the same r. Surprisingly, though, the rate at which a gyroscope carried on the orbiting body precesses takes exactly the same form as in the Newtonian case, so we have:
ωs2 = M / r3
and aφ = – M / r3
The Schwarzschild solution has the same perfect spherical symmetry as the gravitational field around a spherical body in Newtonian gravity. However, relativistic effects mean that we can’t assume, as we did in the Newtonian case, that az = aφ; the fact that the station is moving in the φ direction breaks the symmetry. What we can do is use the spherical symmetry to note that an orbit inclined with respect to the station’s orbit will have an identical period, and so we can use the same argument as in the Newtonian case to connect az to ωorbit2, giving us:
Az = az = – ωorbit2 = – M / [r2 (r – 3M)]
If we trust in Zak’s principle, ar + az + aφ = 0 (which is really the vacuum Einstein equation in disguise), we can now get everything we need:
ar = – az – aφ = M (2r – 3M) / [r3 (r – 3M)]
Ar = ar + ωs2 = 3M (r – 2M) / [r3 (r – 3M)]
This value for ar agrees with an explicit calculation of the Riemann curvature tensor in a suitable basis. Note that ar and Ar are greater than the Newtonian values.
The ratio of net accelerations is:
Ar / Az = – 3 (1 – 2M/r)
which will lie between –3, for very large orbits, and –1 for the smallest possible orbits at r=3M, with a value of –2 for the smallest stable orbits at r=6M. So if we measure a ratio of tidal accelerations other than the Newtonian value of –3, that tells us immediately that we are in a relativistic orbit of some kind, without any need to make external observations.
To confirm that r=6M is the transition between stable and unstable orbits, as we claimed earlier, we can compute:
ωr2 = 4 ωs2 – Ar = M (r – 6M) / [r3 (r – 3M)]
Because ωr is slower than the orbital angular frequency ωorbit by a factor of √(1 – 6M/r), the periastrons of a non-circular orbit (the points closest to the central body) will occur after the orbiting body has completed more than one orbit, and so these points will advance around the central body in the same direction as the orbit.
We can cross-check this value by noting that the effective potential for a particle moving in the Schwarzschild geometry, with the same angular momentum as it would have in a circular orbit of radius r0, is [1]:
V(r) = (1/2) (1 – 2M/r) (1 + M r02 / [r2 (r0 – 3M)])
The first two derivatives of V(r), evaluated at r=r0, are:
dV(r)/dr|r=r0 = 0
d2V(r)/dr2|r=r0 = M (r0 – 6M) / [r03 (r0 – 3M)]
This tells us that for r > 6M a particle perturbed slightly from a circular orbit at r=r0 will experience oscillations with an angular frequency whose square is equal to this second derivative.
The Kerr geometry [2] describes a black hole with angular momentum, which is usually parameterised by a quantity, a, that lies between –M and M. We’ll define the positive direction to be whichever way our station is orbiting, so a > 0 means the station is in a “co-rotating” orbit, and a < 0 means it is in a “counter-rotating” orbit, with respect to the black hole’s angular momentum. We will also take it as given that our station is orbiting in the black hole’s equatorial plane, the plane orthogonal to its angular momentum vector.
We’ll use a generalisation of the Schwarzschild coordinates known as Boyer-Lindquist coordinates. The Boyer-Lindquist r coordinate no longer corresponds to the circumference of circles divided by 2π, but we’ll see shortly that it does have one very nice property.
The event horizon is located at r = M + √(M2 – a2). For general values of a, the locations of the smallest orbit and the smallest stable orbit are complicated expressions, but for the extremal values a=±M they become simple: for a=M there are stable orbits all the way down to the event horizon at r=M, while for a=–M the smallest stable orbit is at 9M, and the smallest orbit at 4M.
The nice property of r that we alluded to is that the rate at which a gyroscope carried on the station precesses still takes exactly the same form as in the Newtonian case, so we again have:
ωs2 = M / r3
and aφ = – M / r3
The proper angular velocity for the orbital motion is given by:
ωorbit2 = M / (r d)
where d = r (r – 3M) + 2 a √(M r)
Because the space-time is no longer spherically symmetrical, there is no guarantee that orbits inclined to the equatorial plane will be circular, or even form closed curves, let alone possess the same period as orbits of the same size lying in the plane. So the link we used previously between az and ωorbit no longer applies, and we need to derive az from first principles: by computing the Riemann curvature tensor in a suitable basis, to find all the tidal accelerations. The results are:
Az = az = – M [r2 – 4 a √(M r) + 3 a2] / (r3 d)
ar = M [r (2r – 3M) – 2 a √(M r) + 3 a2] / (r3 d)
along with aφ which we’ve already noted. If a > 0, ωz (defined by ωz2 = –az) will be less than ωorbit, and the nodes where an ocillating test particle crosses the plane of the unperturbed equatorial orbit will be separated by more than 180 degrees; if a < 0 then ωz > ωorbit, and the nodes will be separated by less than 180 degrees.
We also have:
Ar = 3M [r (r – 2M) + a2] / (r3 d)
Ar / Az = – 3 (1 – 2 [a – √(M r)]2 / [r2 – 4 a √(M r) + 3 a2])
ωr2 = 4 ωs2 – Ar = M [r (r – 6M) + 8 a √(M r) – 3 a2] / (r3 d)
We can re-express the quantity d that appears in the denominators of Ar, Az and ωorbit in terms that include the ratio Ar / Az:
d = 3 (1 + Ar / Az) (a2 + r (r – 2M)) / (2 Ar / Az)
From this, we can see that if Ar / Az = –1, d = 0, and ωorbit becomes singular. So the value of –1 for this ratio marks the smallest possible circular orbit for a given black hole; this is a limiting case that is only possible for orbiting photons. As we’ve previously noted, orbits become unstable when Ar / Az = –2.
In the first section of this page, we discussed the fact that test particle motions on their own only tell us a single dimensionless number, and now that we’re considering orbits around a rotating black hole, which are parameterised by two dimensionless numbers (r/M, the size of the orbit, and a/M, the angular momentum of the hole), the consequences of that become clear: without an external observation, we can never hope to determine both r/M and a/M.
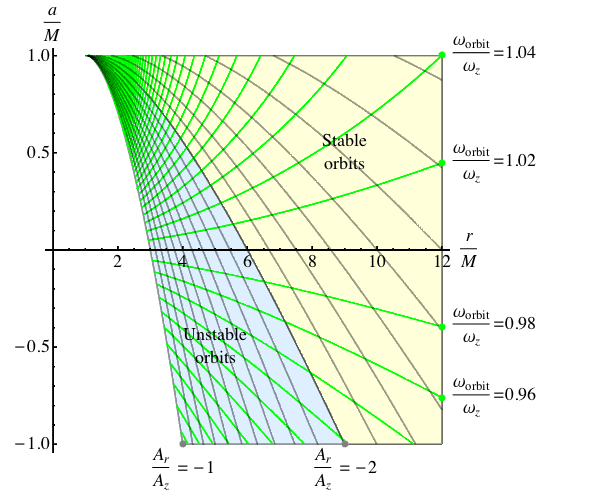
In the diagram below, each point represents a possible circular orbit around a rotating black hole, with r/M and a/M shown on the coordinate axes. If we only know about the motions of test particles inside the station, we can only determine a single dimensionless ratio, such as the ratio Ar / Az. The grey curves are curves of constant Ar / Az, and knowing which one the station lies on will tell us how close the orbit is to the transition from stability to instability, but by itself it can’t fix the values of r/M and a/M. However, by making an external observation that reveals the ratio of the station’s orbital frequency, ωorbit, to the frequency of a test particle oscillating back and forth across the orbital plane, ωz, we can identify which green curve the station lies on, and deduce r/M and a/M from its intersection with the grey curve determined by Ar / Az.

[1] Gravitation by Charles Misner, Kip Thorne and John Wheeler, W.H. Freeman, San Francisco, 1973. Adapted from equation 25.16; what we use as V(r) is half the square of Misner, Thorne & Wheeler’s V(r), in order to get the oscillation rate from the second derivative by making a precise analogy with a non-relativistic particle in a potential well.
[2] Misner, Thorne & Wheeler, op. cit., Chapter 33.
|
|
|